Postgraduate research opportunities in Systems, Dynamics and Control
Postgraduate research opportunities
Postgraduate research at the centre
The centre, as part of Mathematics and Computer Science at Exeter maintains an international reputation for research and we continue to invest in top-quality academics and offer a range of projects to research students to enhance this expertise. For more details of our facilities and training programmes, see our pages for postgraduate taught and postgraduate research degrees.
Apply for a research degree at the centre
Current funded postgraduate research studentship opportunities are listed on our studentships webpage and some ideas for PhD projects are listed below. If you are interested in doing a PhD within the centre, do please contact potential supervisors to discuss possible projects and apply online.
Visitors to the centre
We regularly host visiting researchers of all levels to the centre for stays of a few days to a few weeks. If you are interested in spending some time at the centre, contact a potential host and discuss this with them. Please note the David Rees fellowship offers opportunities to fund visits to mathematics at Exeter.
Examples of PhD research projects
The information below shows some of the PhD research projects which we offer. Please see our academic staff profiles for more suggestions of possible research projects:
Topics in Diophantine Approximation
Diophantine Approximation is the branch of Number Theory primarily concerned with understanding how well we can approximate real numbers by rationals and variations on this theme. In Metric Diophantine Approximation we are interested in understanding, from various perspectives, the "size" of certain sets of "well-approximable points". In the Euclidean setting, fundamental results of Khintchine and (respectively) Jarnik provide neat characterisations of the Lebesgue measure and Hausdorff measure of the classical sets of well-approximable points. Nowadays there is great interest in extending these results beyond the classical setting in innumerable possible directions. Such extensions draw on ideas and techniques from a variety of areas including, but not limited to, Fractal Geometry, Dynamics, Ergodic Theory, and Number Theory.
Find out more about Demi's research on her personal website.
Mathematical modelling of human behaviour and performance
Prof Krasimira Tsaneva-Atanasova
A human operator’s emotional state has been highlighted as an important factor in safety critical industries, and research has shown that high levels of acute stress are often a source of errors. Attentional Control Theory suggests that these errors in decision-making and motor skill performance in stressful environments are due to impaired attentional control and visual information pick-up; driven by an imbalance between goal-directed and stimulus-driven attentional networks. In cognitive systems the laws governing such multi-faceted human behaviour are part of the characteristic phenomenology of complex systems. This project therefore seeks to apply mathematical modelling techniques to behavioural data (an objective measure of attentional control), in order to determine particular signatures of effective responses to stress and their relationship to performance. This analytical approach will be applied to a number of stressful environments and tasks such as flight navigation, surgery, military, and sport.
For further information please email Dr Krasimira Tsaneva-Atanasova.
Mathematical modelling of signalling pathways in biological systems
Prof Krasimira Tsaneva-Atanasova
There is a growing need for applications of our understanding of signal transduction and cellular regulation in biotechnology and health care. Dynamic mathematical modelling and analysis combined with simulation of signal transduction pathways is an important step toward meeting this need. In silico experiments, i.e. model-based computer simulations of the most complex biological systems are much cheaper and faster than laboratory experiments. Simulation scenarios can be precisely formulated and made comprehensible. In addition, in silico experiments help avoid ethically questionable animal experiments. The project will involve close interplay between experimental investigation of pathways and the mathematical representations of molecular, cellular and network dynamics that enables the understanding of complex biological functions such as behaviour. The PhD student will develop and analyse dynamical systems models of signalling pathways using the qualitative theory of differential equations in combination with new data mining methods.
For further information please email Dr Krasimira Tsaneva-Atanasova.
Synchronization and chimera states
Neural systems composed of a network of almost identical cells, each of which has comparatively simple dynamics. One of the fundamental problems in neurophysiology is to understand how such a system can self-organize itself into a functioning system. This PhD project will look at some aspects of frequency synchronization and unusual attractors such as “chimera states” (Abrams and Strogatz, Phys Rev Letts 93:174102, 2004) that have recently been discovered in such systems. This project will proceed by a combination of theoretical and analytical studies, combined with numerical simulations to build models of and understand the features of the cells, the coupling, the network structure and bifurcations that give rise to such states.
Dynamics and number theoretic aspects of piecewise isometries
Dynamical systems with discontinuities and lack of hyperbolicity are poorly understood in many cases, partly because there are many ways in which discontinuities can appear, and partly because most techniques in dynamical systems use hyperbolicity in a fundamental way. For a particular class of such systems (piecewise isometries) there are fundamental problems that remain unsolved, relating to the existence and geometry of periodic trajectories. This project, supervised by P. Ashwin and N. Byott, will use geometric and algebraic number theory methods to try to answer some of these questions and in particular to study the combinatorial structure of dynamically induced packings of phase space.
Networks of Dynamical Systems
The behaviour of more complicated dynamics systems in a variety of applications can be usefully broken down into an interplay between network structures and node dynamics. Of particular interest is how synchrony and symmetry breaking can organize the behaviour of such systems.
Causodynamics of waves and patterns in reaction-diffusion systems
The project will be concerned with numerical solution of partial differential equations of 'reaction-diffusion' type, particularly of the types that are used to describe waves and patterns in mathematical biology. Specifically, the project will focus on sensitivity of such solutions to initial conditions and/or perturbations. This sensitivity will be determined by the method of 'causodynamics', which involves integration of the adjoint linearized equations backwards in time. A few selected problems will be considered during the project. The tentative plan of the research is a follows. At the initial stage, this will be Turing patterns and propagating pulses in one spatial dimension and spiral wave solutions in two spatial dimensions. At the next stage, spatio-temporal chaos (such as generated by Kuramoto-Sivashinsky equation) in one spatial dimension will be considered. Further examples will concentrate on more complicated regimes, relevant for modelling cardiac fibrillation, such as: competing spiral waves, 'mother rotor' regimes, and two- and three-dimensional spiral and scroll wave turbulences of various sorts. The overall aim is to characterize the possibilities to control such regimes by small perturbations. The project will require from the candidate some fundamental mathematical knowledge, including linear algebra, basic dynamical systems theory, asymptotic methods for ordinary and partial differential equations, and possibly some elements of functional analysis. It will also require suitable IT skills, including numerical solution of partial differential equations. The candidate should be prepared to learn necessary disciplines and skills during the project, if they do not possess them already.
Hybrid asymptotic-numerical methods for cardiac excitation models
Modern mathematical models of cardiac excitation are ''stiff'', in that they involve processes happening on time scales from fractions of a millisecond to tens of seconds and longer, which in conjunction with the spatial complexity of the heart, makes cardiac simulations a serious computational challenge. On the other hand, the time scale ratios can be used as small parameters in asymptotic methods. One possible application for such asymptotics is their use in large-scale computations of normal and abnormal cardiac excitation patterns, in hybrid asymptotic-numerical schemes. The proposed Ph.D. project will make first steps in that direction, based on the recent progress by the supervisor's group in cardiac asymptotics. The main goal will be a methodology to combine asymptotic description of excitation waves, in terms of propagating fronts, with the original partial-differential equations for spatio-temporal evolution of nonlinear dynamic fields. This will be done first in one spatial dimension and subsequently extended to two and three dimensions. Initially we will consider simple topologies, when the front is a point (in one spatial dimension), or a manifold without internal borders (in two and three spatial dimensions). Then we extend it to the case of wavebreaks, where the wave front has edges within the excitable medium. Finally, we shall consider extension from the initial modomain (semi-parabolic PDE systems) to bidomain (with added elliptic equations) models of the heart.
The project will require knowledge of mathematical biology, asymptotic and numerical methods for PDEs and software development. Hence, depending on their background, the candidate may be required to do relevant modules offered by this and/or other Departments in this University, to be specified by the supervisor, and pass them at least at the level acceptable by M.Sc. standard, in his/her first year of the project.
Coevolution of bacteria and phages
Interaction of predators and prey can lead to a variety of complicated behaviours, particulary in the spatially extended context. For instance, theory predicts "pursuit-evasion" waves, where the only chance for prey to flourish is to flee away from the predators, and vice versa. The proposed project will look at the problem of coevolution of prey (bacteria) and predators (phages) as pursuit-evasion waves in the "trait space" instead of the physical space. This view has been motivated by in-vitro experiments when evolution bacteria and phages happens under controlled conditions and in real time. The project will involve construction and numerical and analytical studies of mathematical models of such coevolution, with a view to identify relevant scales of parameters and possible ways to relate the models with the experimental data.
The project will require knowledge of mathematical biology, dynamical systems theory and asymptotic and numerical methods for PDEs. Hence, depending on their background, the candidate may be required to do relevant modules offered by this and/or other Departments in this University, to be specified by the supervisor, and pass them at least at the level acceptable by M.Sc. standard, in his/her first year of the project.
Iterative methods of calculation of response functions of spiral waves
Spiral waves are a form of self-organization observed in distributed active media, such as some catalytic chemical reactions or heart muscle. Spiral waves in heart underlie dangerous arrhythmias. A peculiar feature of spiral waves is "wave-particle duality": being waves, they behave like particles when drifting in response to generic small perturbations. This allows description of their drift in terms of ordinary differential equations of motion, which are easier to solve and more amenable to qualitative analysis than the "reaction-diffusion" nonlinear partial differential equations of the original models. The success of this asymptotic approach depends on knowledge of so called response functions, which is critical eigenfunctions of the adjoint linearized operator. A numerical method for calculating the response has been developed recently by the supervisors' group, and its workability has been successfully demonstrated on a number of concrete models, including models of cardiac tissue. However, this method involves direct LU decomposition of a matrix of linearization, which severely limits the achievable spatial resolution, due to memory demands.
The subject of the present project will be development and investigation of alternative methods of calculation of response functions. The methods are likely to be iterative and based on the Conjugate Gradients idea. Apart from the development of the methods, the proposed programme will include study of their convergence and computational efficiency, and test applications to dynamics of spiral waves in selected models, most likely from cardiac dynamics.
The project will require knowledge of mathematical biology, asymptotic and numerical methods for PDEs and software development. Hence, depending on their background, the candidate may be required to do relevant modules offered by this and/or other Departments in this University, to be specified by the supervisor, and pass them at least at the level acceptable by M.Sc. standard, in his/her first year of the project.
Statistical properties of strange non-chaotic attractors
Chaotic systems driven by quasiperiodic maps can give rise to strange non-chaotic attractors: attractors with complex fractal structure but zero Lyapunov exponents. Such attractors have been observed numerically in many systems, but many rigorous results are yet to be obtained. Dynamical questions concern those of statistical/ergodic properties, fractal structure, and robustness under perturbation.
Noisy intermittent dynamical systems
Chaotic maps withe neutral (non-hyperbolic) fixed points display bursty intermittent phenomenon: periods of chaos interspersed with long stretches of regular dynamics. Dynamical questions are raised for multidimensional intermittency maps and the qualitative behaviour of such maps with the addition of noise. These maps are known to be useful for generating time series data with long-memory and applications include modelling queue and network behaviour.
Extreme Value Theory and Risk Analysis
For a sequence of random variables X_1,..X_n, extreme value thoery (EVT) is a study of the limiting distristibution of max(X_1,..X_n) (or some intermediate order) up to some linear scaling. A possible research project is to study EVT in the context of deterministic dynamical systems, where the sequence X_1,..,X_n can be viewed as an iteration generated from a deterministic map. Applications of EVT include climate systems, stochastic systems and finance.
Theory of dynamical systems with delay
When a dynamical system is modelled by a differential equation with delayed arguments (see the famous Mackey-Glass equation for an example) the dimension of the phase space (that is, the space of possible initial conditions) is infinite-dimensional. That's why even a scalar delay differential equation (DDE) can show chaos with many unstable Lyapunov exponents.
While the basic theory for systems with constant delay is well settled, systems in which the delay depends on the state still offer many challenging open problems. One challenging project is the study of so-called homoclinic connections (orbits converging to an equilibrium forward and backward in time) and their relations to periodic orbits. At the moment there is no rigorous theory extending the classical theory for ordinary differential equations to DDEs with state-dependent delay. The source of the problem is that the time-t map for DDEs with state-dependent delay is only differentiable once (at least this only this could be proved until now).
Another open problem is the persistence and regularity (smoothness of degree larger than one) of invariant manifolds. Solving this problem would permit the full generalization of center manifold theory for ordinary differential equations, and thus, all of the classical local bifurcation theory could be applied to DDEs with state-dependent delays.
Tracking bifurcations in experiments
The introduction of a stabilising feedback control loop into a physical experiment with nonlinearities enables "equation solving" directly on the experiment. A feedback loop takes an output y(t) of the experiment, subtracts it from a reference signal r(t), and feeds back this difference y(t)-r(t) back into the experiment (processed and amplified by so-called control gains).
The approach taken here is to adjust r(t) iteratively (ideally in a Newton iteration) to make y(t)-r(t) identically zero. In this way, one uses the feedback loop to observe trajectories of the uncontrolled system. The advantage of this at first sight cumbersome approach is that the dynamical stability of the uncontrolled trajectory does not matter.
The long-term goal is to develop a set of tools equivalent to AUTO for experiments. A major obstacle in practical experiments is that, comparted to numerical calculations, the disturbance are much larger. Thus, many of the classical algorithms for finding and tracking bifurcations (say, saddle-node or Hopf bifurcation) have to be re-developed.
Collaborators in Bristol and Plymouth have set up flexible mechanical and electrical experiments where new ideas for algorithms can be tested.
Banner image references
-
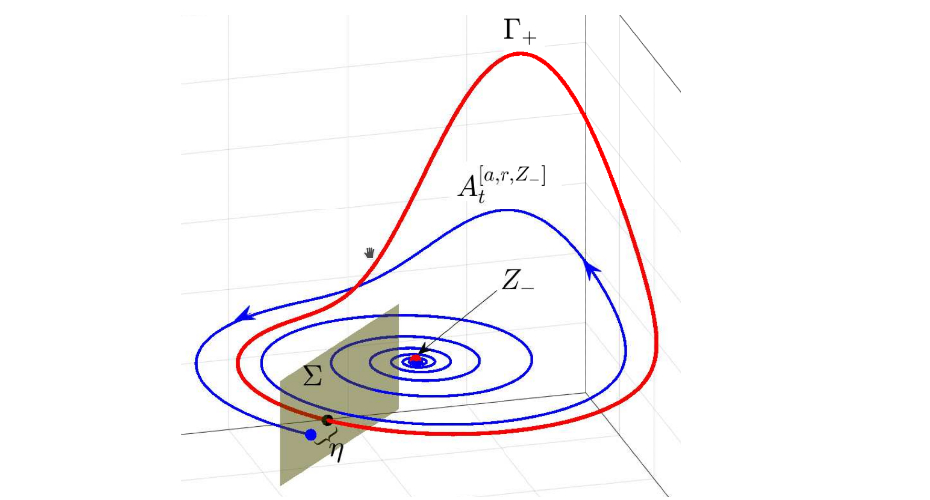
Image 1 - Loss of tracking in non-autonomous Roessler system (Alkhayuon, Ashwin 2020)
-
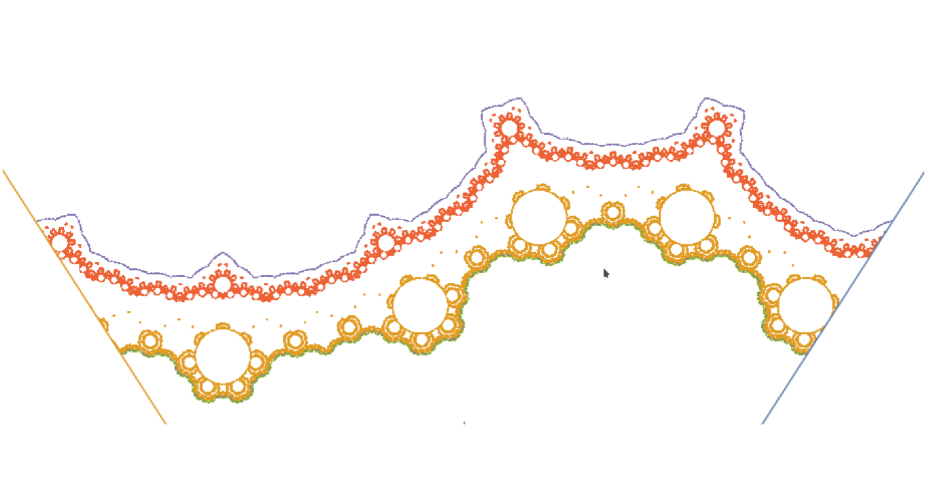
Image 2 - Orbits of 5 points for piecewise isometry (Ashwin, Goetz, Perez, Rodrigues 2018)
-
Image 3 - Forecasting error for Lorenz system (Sterk, Holland 2018)